Myth 1: Springs Can Be Compressed Indefinitely Without Damage

Reality: Every spring has a limit to how much it can be compressed (for compression springs) or stretched (for extension springs) without suffering damage. This limit is often defined by the spring's maximum deflection travel (for compression springs) or maximum deflection travel (for extension springs). Compressing a spring beyond its maximum travel or stretching it beyond its maximum extension travel can cause deformation, where the spring does not return to its original shape, leading to permanent damage and loss of load.b

The amount of force a spring can withstand before reaching its solid height is determined by several factors, including the wire diameter, coil count, and material type properties. For example, a spring with a thicker wire diameter may have a higher solid height limit than one with a thinner wire. However, even springs made from robust materials like music wire or chrome silicon can suffer damage if compressed beyond their design specifications.

To determine the solid height of your spring, you must first decide on the type of ends your spring requires. The most common and cost-effective option for compression springs is the closed and squared ends. These ends provide a good balance between stability and simplicity. However, if your spring needs to rest precisely flat on a surface without the small amount of wobble that closed and squared ends might introduce, you should consider closed and ground ends. Keep in mind that closed and ground ends typically increase the cost of the spring, so it's important to confirm that this feature is necessary for your application. Typically a good rule of thumb to determine if you need closed and ground ends is the spring index. If your spring has and index of between 3 to 5.5 index then you should have closed and ground ends. Why? Because a small index means your spring has a very tightly wound outer diameter coupled with a thick wire diameter meaning the spring will probably not stand up straight vertically without closed and ground ends. 

Example of a tightly wound small index spring with closed and ground ends

If your spring design involves threading the ends into another component, open ends might be the right choice. For situations where your spring needs to be threaded onto a screw or device that requires stability at both the top and bottom, you may need multiple closed ends, such as double or more closed ends. This configuration ensures that the spring remains securely in place while providing the desired functionality.

For compression springs with closed and squared ends, the solid height (SH​) can be calculated as:

Total Wires = Total Coils + 1

Solid Height = Total Wire * Wire Diameter

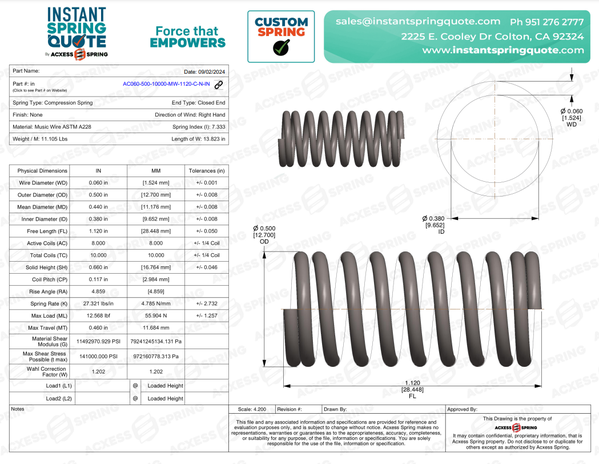
To demonstrate the application of these formulas, we'll reference part number AC060-500-10000-MW-1120-C-N-IN throughout our discussion. By consistently using this specific part number, we can maintain clarity as we explore this critical concepts and their real-world applications. This method will allow us to see how these concepts influence the performance, fit, and reliability of springs, providing a practical understanding of their importance in various scenarios.

Now, let's calculate the solid height for part number AC060-500-10000-MW-1120-C-N-IN. This will give us a clear understanding of the spring's maximum compressed length and how it will perform in its intended application.

Calculation:

Total Wires = Total Coils + 1

Solid Height = Total Wire ⋅ Wire Diameter

1. Calculate Total Wires

Total Wires = 10 + 1 = 11

2. Calculate Solid Height 

 Solid Height = 11 ⋅  0.060 inches

Solid Height = 0.660 inches

The solid height (SH) of the compression spring with 10 total coils, a wire diameter of 0.060 inches, and Closed & Squared ends is 0.660 inches. This formula indicates that once the spring is compressed to its solid height of 0.660 inches, further compression will cause the coils to contact each other, leading to potential deformation or damage.

Clarification: To avoid damaging your springs, it's essential to operate them within their specified maximum deflection travel  limits. Acxess Spring's Online Spring Force Tester can help you determine the maximum load and maximum deflection for your springs, ensuring they are used within safe limits and maintain their functionality over time. By calculating and testing these limits, you can prevent deformation and extend the life of your springs.

Myth 2: Heavier Springs Are Always Stronger

This formula shows that the spring rate increases significantly with the wire diameter (d) and decreases with the number of active coils (N) and the mean coil diameter (D). Therefore, a spring with a smaller wire diameter but optimized design might be stronger than a heavier spring with a larger diameter but poor design. Once again, we’ll use part number AC060-500-10000-MW-1120-C-N-IN to exemplify this principle.

Calculation:

1. Calculate d^4:

d^4 = (0.060)^4 = 0.00001296 cubic inches

2. Calculate D^3:

D^3 = (0.440)^3 = 0.085184 cubic inches

3. Plug these values into the formula:

k = 11,492,970.929 ⋅ 0.00001296 ÷ 8 ⋅ 0.085184 ⋅ 8​

4. Calculate the denominator:

8 ⋅ 0.085184 ⋅ 8 = 5.451776

5. Calculate the numerator:

11,492,970.929 ⋅ 0.00001296 = 148.94890323984

6. Divide the numerator by the denominator:

k = 148.94890323984 ÷ 5.451776 ≈ 27.32117 lbs/in 

The calculated spring rate (k) for the given spring is approximately 27.321 lbs/in. This spring rate indicates the stiffness of the spring, meaning that for every inch of compressed travel, the spring will exert a force of 27.321 pounds.

Myth 3: Springs Only Come in Standard Sizes and Designs

A common myth is that springs are only available in standard sizes and designs, limiting their use in custom or specialized applications. This belief often leads to the misconception that custom solutions are either unavailable or prohibitively expensive, which can deter engineers and designers from exploring innovative spring designs.

Reality: While standard-sized springs are widely available and suitable for many applications, custom springs can be designed to meet any specific need. Custom springs can vary in size, shape, material, and load capacity, allowing them to be tailored to unique applications. For example, in the medical field, small springs are often used in intricate devices, requiring precise dimensions and specific performance characteristics. In the world of spring calculation there are over 70 trillion custom spring designs available in Spring Creator 5.0 meaning the possibilities are endless in spring design. 

Custom spring design involves determining the necessary dimensions and characteristics based on the intended load and travel deflection requirements. For instance, the wire diameter (WD) of a spring is a crucial factor in determining the spring's strength and stiffness. The wire diameter can be calculated using the following formula:

WD = (OD - ID) ÷ 2 

Where:

• OD is the outer diameter of the spring.

• ID is the inner diameter of the spring.

For example, if a custom spring design specifies an outer diameter of 0.500 inches and an inner diameter of 0.380 inches, similar to the dimensions of part number AC060-500-10000-MW-1120-C-N-IN, the wire diameter would be calculated as:

WD = 0.500 inches − 0.380 inches ÷ 2

WD = 0.120 inches​ ÷ 2

WD = 0.060 inches

This calculation determines that the wire diameter should be 0.060 inches to meet the specified outer and inner diameters.

Understanding and calculating the wire diameter is essential because it directly impacts the spring's overall performance, including its load capacity, spring rate, and deflection. Properly selecting the wire diameter ensures that the spring will function correctly within its intended application, whether it’s designed for high load-bearing capacity or for precise deflection under lighter loads.

Innovative Design Support: Designing a custom spring does not necessarily mean high costs or long lead times. Advances in spring manufacturing and design software, such as Acxess Spring's Spring Creator 5.0, have made it easier and more cost-effective to create custom springs. Spring Creator 5.0 allows engineers to input specific requirements and instantly generate a detailed design. This tool enables visualization and refinement of the spring design before production, ensuring the final product meets all specifications. By using Spring Creator 5.0, you can push the boundaries of spring innovation, creating solutions that perfectly meet the unique needs of your applications.

Myth 4: Spring Material Doesn’t Affect Performance

Reality: The material type of a spring is crucial to its performance, especially in extreme environments. Different materials have different properties that make them more or less suitable for specific conditions. For instance:

• Stainless steel ASTM A313 is highly resistant to corrosion and is ideal for use in humid or salty environments, such as marine applications.

• Music wire ASTM A228 offers excellent tensile strength, but may not be suitable for corrosive environments unless properly coated.

• Chrome silicon is often used in high-temperature applications because of its superior heat resistance.

Choosing the right material ensures that the spring maintains its strength, elasticity, and resistance to environmental factors like corrosion, temperature changes, and wear. The material selection directly affects the spring's ability to handle stress and deflection without failure.

Clarification: When designing springs for extreme environments, it's essential to choose the right spring wire material. Acxess Spring's Knowledge Base provides extensive information on material selection, helping you understand the properties of different materials and choose the best one for your specific application. This resource, combined with the material selection features in Spring Creator 5.0, ensures that your springs will perform reliably even in the most challenging conditions.

Myth 5: Springs Are Simple and Don't Require Specialized Knowledge to Design

Calculation: 

The torque (T) can be calculated using the formula:

T = k ⋅ θ

1. Plug in the values:

T = 0.00212 in-lbs./deg. ⋅ 90 degrees

T = 0.1908 in-lbs.

The torque (T) for the torsion spring with a spring constant of 0.00212 in-lbs./deg. and desired travel of 90 degrees is 0.1908 in-lbs.

This formula helps determine whether the spring will provide the required torque without deforming. 

Solution: For those who might not have extensive experience in spring design, or even for seasoned engineers looking to refine their knowledge, Acxess Spring's Knowledge Base is an invaluable resource. This comprehensive list of articles offers a wealth of information on all aspects of spring design, from the basic principles to advanced techniques. It includes detailed guides, practical examples, and troubleshooting tips that can help you avoid common mistakes and optimize your designs.

By utilizing Acxess Spring's Knowledge Base, you can gain the specialized knowledge needed to design springs that meet your application's requirements, ensuring both performance and reliability. Whether you're new to spring design or looking to deepen your expertise, this resource is the key to making informed decisions and avoiding the pitfalls of poorly designed springs.

Empowering Spring Design

Understanding the realities of spring performance and design is crucial for making informed decisions and avoiding costly mistakes. By debunking these common myths, we hope to improve your understanding of springs and their applications. Whether you're working with standard or custom springs, selecting the right materials, designing within performance limits, or utilizing advanced tools like Spring Creator 5.0, the Online Spring Force Tester, and Spring Finder, you can ensure that your springs will perform reliably and meet your specific needs.

Springs may seem simple at first glance, but their design and application require careful consideration and expertise. By recognizing and overcoming these misconceptions, you can make better decisions that will enhance the performance and longevity of your springs, leading to more successful outcomes in your projects. Leveraging the powerful tools from Acxess Spring will not only help you design and test springs more effectively, but also push the boundaries of what is possible in spring technology, ensuring your solutions are both innovative and reliable.